On March 20, 2023, a team of four researchers announced that they had discovered a shape. This was big news in the world of mathematics and was widely reported in the media.
The reason for the excitement was that this shape is an einstein (in German, ‘ein stein’ means “one stone”), an object that mathematicians had been searching for almost 60 years. An einstein – also known as an aperiodic monotile – is a shape that can tile a surface plane, but only in a non-repeating manner. The shape they had discovered looked like a hat, and simple enough that anyone could draw it.
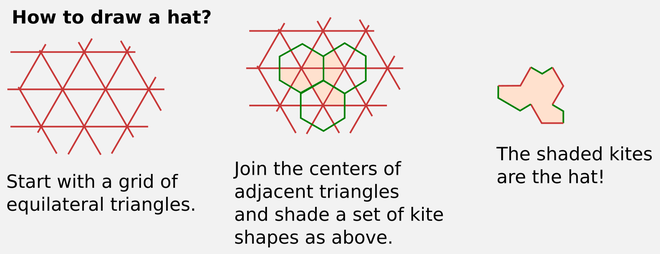
To tile a surface with a set of shapes means to cover it with copies of those shapes, with no gaps and no overlapping. A tiling in which one is not allowed to use the reflections of the shape is called a chiral tiling. A chiral einstein is a single shape that tiles the plane but only aperiodically and chirally. The tiling by the hat uses both the shape and its reflection. So the hat is an einstein, but not a chiral einstein.
On May 28, the same team announced the discovery of a shape called a spectre which was a chiral einstein. To get a spectre, we start with a straight-edge polygon called Tile(1,1) that looks similar to a hat, and add wiggles to its sides.

Most tilings, such as the ones we see on bathroom walls, beehives, or wallpapers, are repeating patterns. This means if you move the pattern a little to the left or right, or rotate by a certain angle, you should see the same shape.

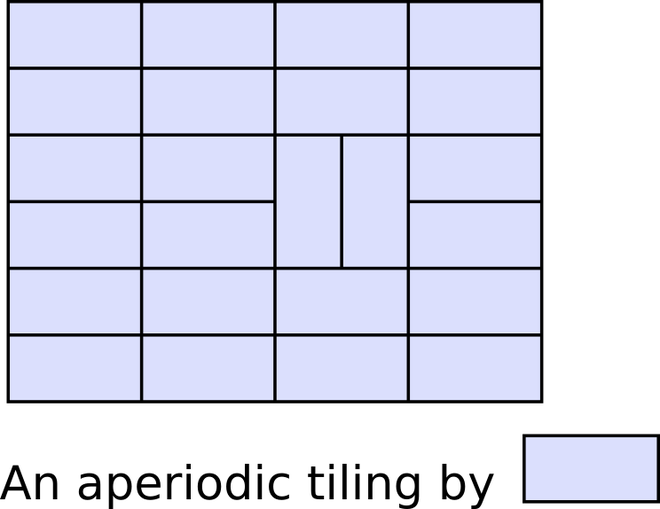
Aperiodic tilings are those whose shapes don’t repeat themselves. In the example of rectangles on the bottom (above), the plane is tiled by rectangles, one of whose sides is twice as long as the other. In this tiling, the shorter side is the vertical side for all but a pair of rectangles. So if we shift the pattern, these two aberrant tiles are not going to coincide with any other tile.
However, the surface itself can be tiled periodically with this rectangle, so it’s not an einstein.
In fact, it is surprisingly hard to come up with tile sets that tile the plane but only aperiodically. The first such set, discovered in the 1960s, consisted of tiles of 20,426 different shapes! In 1974, the mathematician (and later Nobel laureate) Roger Pensrose discovered a set of just two tiles that could tile a plane aperiodically. Since then, mathematicians have been in search of an einstein – the one stone.
David Smith, a shape-hobbyist from England, was obsessed with finding an einstein. He tinkered around with shapes and used an online geometry platform to check whether they produced short-range tilings. After a decade of trying, in October 2022, he hit upon a 13-sided polygon that seemed to work. This was the hat.

To show that the hat was an einstein, one has to prove that the tiling extends aperiodically to the whole plane and that there is no periodic tiling anywhere. Mr. Smith did this with the help of computer scientist Craig S. Kaplan, software developer Chaim Goodman-Strauss, and mathematician Joseph Myers. Their work was the March 2023 paper.
While the researchers were still working on the proof of the hat being an einstein, Mr. Smith stumbled upon another einstein. This one looked like a turtle and was also 13-sided. The finding prompted the group to wonder if there are many more aperiodic monotiles lurking around.
That’s how they found that both the hat and the turtle were members of a continuous family of shapes, all of which tile the plane in the same way. Mr. Kaplan’s webpage has an animation that moves smoothly through tilings by this family of shapes. The figure below shows stills from this animation.
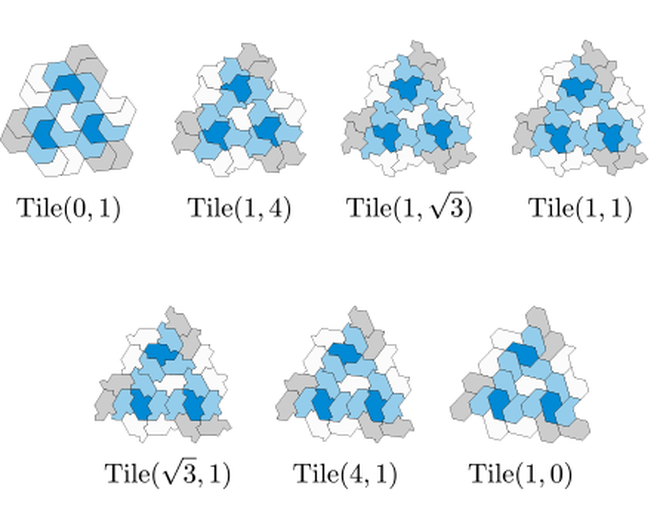
Different members of this hat family have different side lengths. To start with: the hat has two distinct side lengths, 1 and the square root of 3. If we vary the ratio between these two lengths, keeping the angles between the adjacent sides fixed, we get a family of shapes. A member of the family with side lengths a and b is denoted Tile(a,b). The hat is Tile(1,sqrt(3)) and the turtle is Tile(sqrt(3),1). In the March paper, the authors used this family to come up with an innovative proof of the fact that the hat is an einstein.
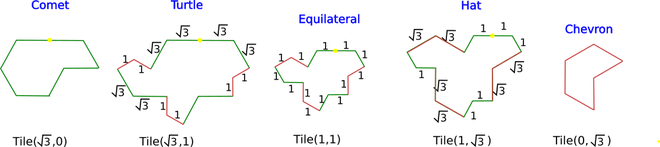
In this family of tiles, Tile(1,1) is special because all its side lengths are equal. It is an equilateral polygon. This in turn means there are more ways in which adjacent tiles can be placed next to each other. In fact, this particular tile, along with its reflection, can tile the plane periodically, which makes it different from all the other tiles in the family Tile(a,b).
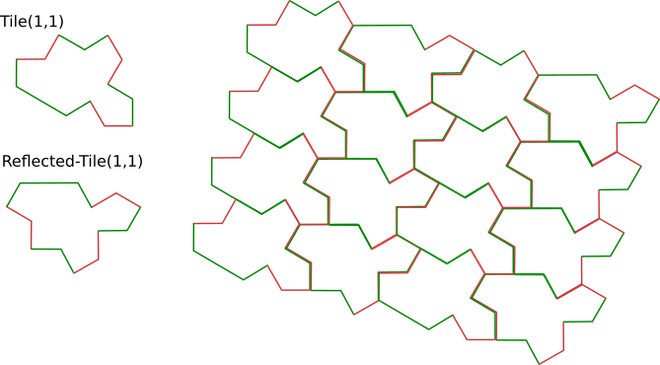
The authors discovered that the wider range of adjacencies allowed by Tile(1,1) had a lucky side-effect. There was a chiral tiling of the plane using Tile(1,1). This observation led to the May 2023 paper.
Like most developments in mathematics, it’s hard to know at the outset whether aperiodic montiles have applications in science. But going by the impact Penrose tilings had on science, these einsteins could also lead to new findings.
In fact, about 20 years after the discovery of the Penrose tiles, they led to the discovery of quasicrystals. The atoms of a crystal are arranged in a periodically repeating pattern, but in quasicrystals, the pattern is ordered but not periodic. The knowledge of Penrose tilings helped scientists make sense of these aberrant structures, leading to more detailed research on quasicrystal structure in materials. Dan Shechtman, who first reported the discovery of quasicrystals in the early 1980s, received the Nobel Prize in chemistry in 2011 for his work.
There could also be more mathematical problems related to the einstein. We could hunt for monotiles with fewer sides. Even with the hat-turtle monotile there is work to be done. For example, mathematicians have found connections between the Penrose tiling and a way to cover a hyperbolic plane with pentagons. Are there similar connections to monotiles?
The discovery of the einstein also tells us that we don’t need to be professional mathematicians to tackle these problems. Anyone who is interested and keeps trying can make the next big discovery!
Sushmita Venugopalan is a mathematician at the Institute of Mathematical Sciences, Chennai.







